Measuring water flow

Using the float method and rating curves

Water often is delivered to aquaculture facilities through open channels. There are several ways to measure flow in open channels, most of which are based on the equation:

Flow Rate (m³ per second) =
Flow (m²) x Velocity (meters per second)

In a straight section of a stream or channel, each depth or stage height corresponds to a unique cross-sectional area and velocity. Thus, by measuring the area and velocity of flow at a number of stage heights, a graph relating stage height to flow rate can be created (Fig. 1). With such a rating curve available, channel flow can be estimated from mea-surements of stage height alone.

Float method

Considerable effort is required to prepare a rating curve. Where it is not necessary to obtain frequent measurements of flow rate in a small stream or channel, the float method can be applied. 

In this procedure, the cross-section area of a straight stretch of channel is determined by measuring the width and obtaining the average depth by sounding. The time required for a float to travel a measured distance in the channel reflects the surface velocity. The average velocity is calculated by multiplying the surface velocity by the appropriate coefficient from Table 1.

Boyd, Velocity coefficients for the float method, Table 1

Average Flow Depth (m)	Coefficient
0.25	0.64
0.50	0.67
0.75	0.70
1.00	0.71
1.50	0.74
2.00	0.76
3.00	0.77
4.00	0.78
5.00	0.79

Table 1. Velocity coefficients for the float method of determining channel velocity.

 

To illustrate the float method, suppose a channel is 8 meters wide and has an average depth of 1.5 meters. The area of flow is 12 square meters (8 m x  1.5 m). A float travels 10 meters in 85 seconds. Thus, the surface velocity is 0.118 millimeters per second (10 m ÷ 85 seconds). The coefficient for 1.5 meters average depth is 0.74, so average velocity is 0.087 millimeters per second (0.118 meters per second x 0.74). The flow rate is 1.04 cubic meters per second (12 m² 0.087 x meters per second). The equation for the float method is:

Weirs

Weirs can be installed in streams or channels to measure flow rate. A weir is a barrier plate that constricts the flow in an open channel through a fixed-size opening. Common shapes of weirs are rectangular, trapezoidal and triangular (Fig. 2).

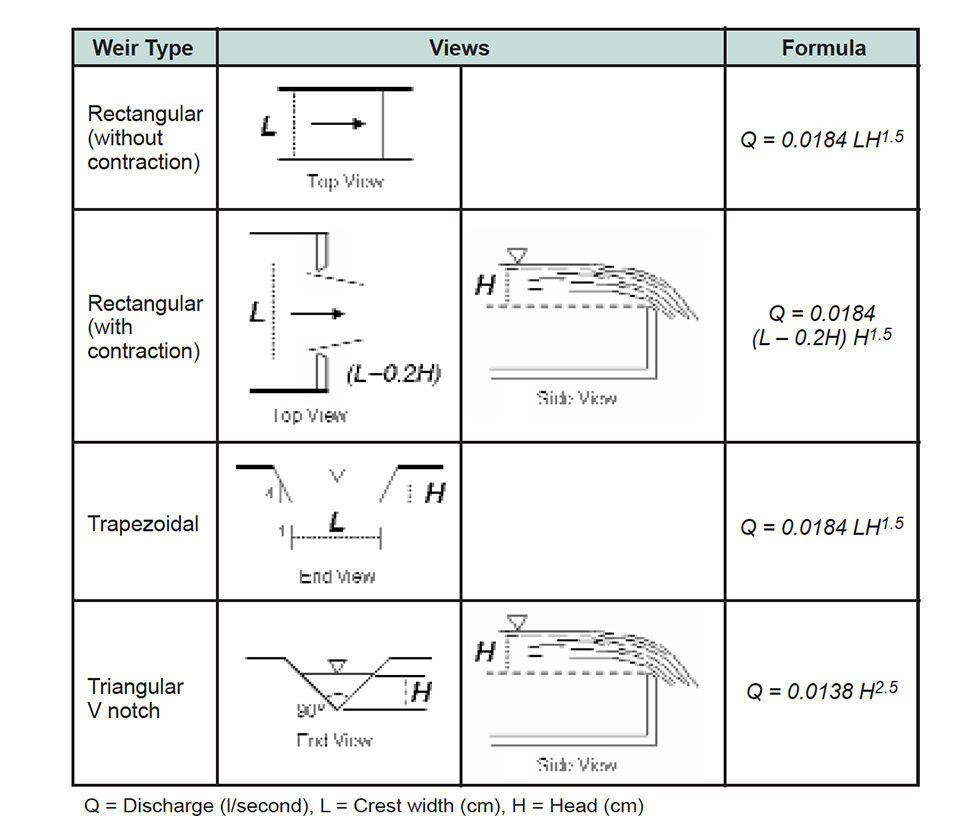
The bottom edge of the opening through which the water flows is the weir crest. The depth of water above the elevation of the bottom of the crest is the head. The head causes water to flow through the crest. The water level must be lower downstream of the weir plate than upstream so there is air flow under the stream of water passing through the weir.

It is important to note the two types of rectangular weirs. The weir crest is the same width as the channel for noncontracted rectangular weirs. In contracted rectangular weirs, the weir crest is not as wide as the channel, and the entering flow is constricted by the ends. 

Triangular V-notch weirs do not have a crest, for the water flows through a notch. These weirs are more applicable than other types for small channels because a greater head is achieved at low flow. The head is the depth of flow measured to the bottom of the V notch. Also, at low heads, water still exhibits free flow over V-notch weirs.

Estimating flow from weirs

The flow from weirs can be estimated with the equations also provided in Fig. 2. Weir plates must be constructed precisely to the specified geometric shapes and installed with the weir crest level. The head must be measured at a point at least four times the head upstream from the crest. This is because the water surface slopes downward as it approaches and flows over the crest. The channel depth between the weir plate and point for head measurement must be at least twice the head.

Flow estimate accuracy

Rectangular weir equations often are used to measure the flow rate of water entering aquaculture ponds through inlet gates. However, these gates frequently do not meet the design requirements of rectangular weirs. 

The accuracy of flow estimates made with weir equations can be evaluated by comparing them to direct estimates of inflow to ponds. The flow rate can be measured directly by lowering the pond water level a few centimeters and estimating the time required to replace the water. 

Rate adjustments

If weir equations do not provide reasonable estimates of flow within 10 percent of the measured values, adjustments are in order. The most reliable way to make the adjustments is to compare equation flow estimates and direct measurements at four or five different head values over the range to be employed during pond water management. 

Weir equations can also estimate flow rates through outlet gates. It is seldom possible to measure discharge directly to check the accuracy of weir equations. Nevertheless, most gates on a farm usually are made to the same specifications, so adjustments for one gate could be used for other gates of the same type.

(Editor’s Note: This article was originally published in the October 2005 print edition of the Global Aquaculture Advocate.)